Biological model II

Download

Flow* biology_II.model

Model description

We present a nine-dimensional continuous model which is adapted from a biological system given in [1]. The modeling ODE is given as below.

    \[ \left\{ \begin{array}{lcl} \dot{x}_1 & = & 3 \cdot x_3 - x_1 \cdot x_6 \\ \dot{x}_2 & = & x_4 - x_2 \cdot x_6 \\ \dot{x}_3 & = & x_1 \cdot x_6 - 3 \cdot x_3 \\ \dot{x}_4 & = & x_2 \cdot x_6 - x_4 \\ \dot{x}_5 & = & 3 \cdot x_3 + 5 \cdot x_1 - x_5 \\ \dot{x}_6 & = & 5 \cdot x_5 + 3 \cdot x_3 + x_4 - x_6 \cdot (x_1 + x_2 + 2 \cdot x_8 + 1) \\ \dot{x}_7 & = & 5 \cdot x_4 + x_2 - 0.5 \cdot x_7 \\ \dot{x}_8 & = & 5 \cdot x_7 - 2 \cdot x_6 \cdot x_8 + x_9 - 0.2 \cdot x_8 \\ \dot{x}_9 & = & 2 \cdot x_6 \cdot x_8 - x_9 \end{array} \right. \]

Reachability settings

We consider the initial set x_1,x_2,x_3,x_4,x_5,x_6,x_7,x_8,x_9\in [0.99,1.01] .

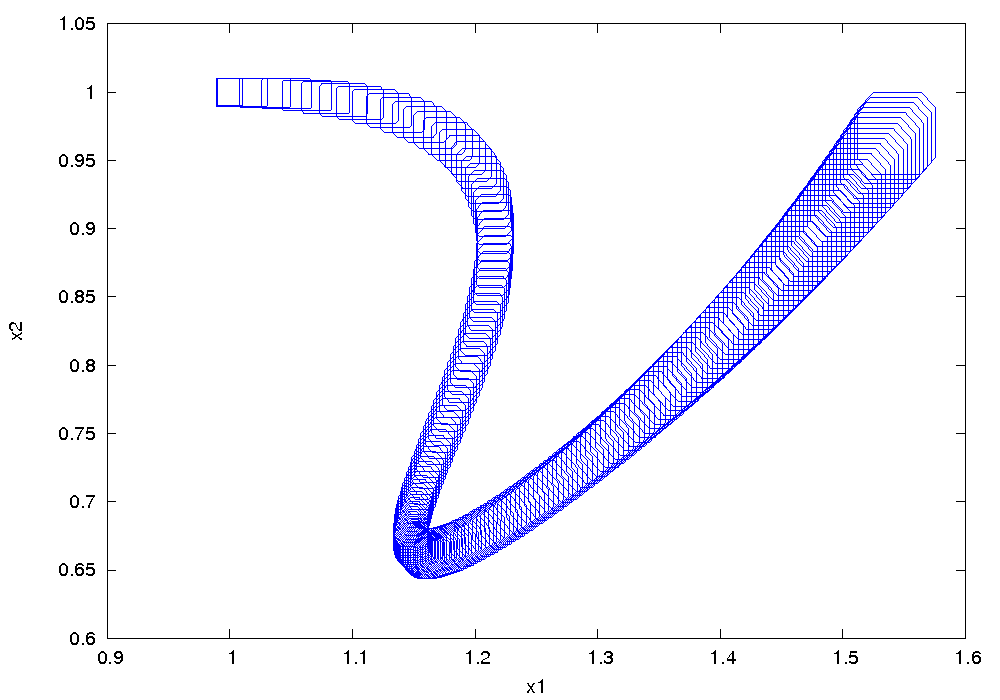
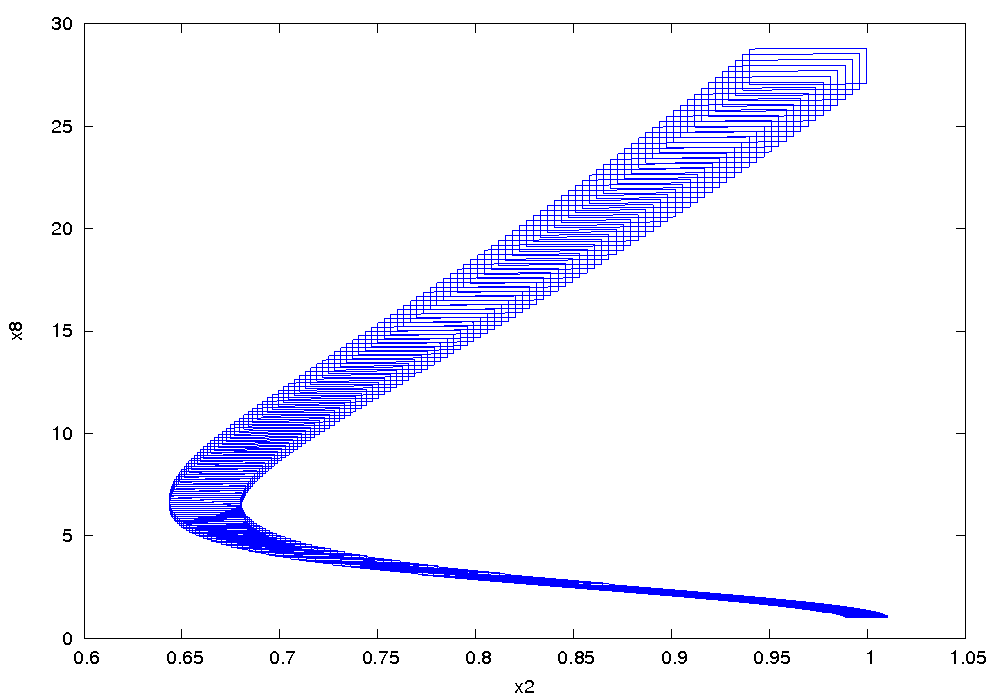
Results

The following figure shows an overapproximation computed by Flow* for the time horizon [0,2] .

biology_II_x1_x2

biology_II_x2_x8

References

[1] E. Klipp, R. Herwig, A. Kowald, C. Wierling, H. Lehrach. Systems Biology in Practice: Concepts, Implementation and Application. Wiley-Blackwell, 2005.